
Hence, the geometric sequence is įinding the sum of the Geometric sequence can be quite difficult. Pick any of them and solve the problems of geometric sequence effortlessly.įind the geometric sequence up to 7 terms if first term(a) = 5, and common ratio(r) = 2. Finally, you have seen two ways to find the terms of GP.The other way to find the various terms in a GP is by substituting the value of n in ar n-1.Keep multiplying the common ratio with the prior term & find the required number of terms. To find the second term, multiply 'a' with the common ratio 'r' a × r.The detailed steps that you need to focus & follow while finding the terms of a GP are listed below:
#ARITHMETIC GEOMETRIC SEQUENCE CALCULATOR HOW TO#
= ar n-1/ar n-2 How to Find the Terms of Geometric Progression?
#ARITHMETIC GEOMETRIC SEQUENCE CALCULATOR SERIES#
Let's consider the geometric series is a, ar, ar 2, ar 3.Ĭommon Ratio(r) = (Any Term) / (Preceding Term) Therefore, the kth item at the end of the geometric series will be ar n-k.
This can be written as b = √ac or b 2 = ac

If a, b, and c are three values in the Geometric Sequence, then “b” is the geometric mean of “c” and “a”.If there are 3 values in Geometric Progression, then the middle one is known as the geometric mean of the other two items.The geometric sequence formula to determine the sum of the first n terms of a Geometric progression is given by:.The nth term of Geometric sequence is a n = ar n-1.The general form of GP is a, ar, ar 2, ar 3, etc., where a is the first term and r is the common ratio.Hence, the Solution of Arithmetic Sequence of 2,4,6,8,10,12,14,16 is 72.0.The list of geometric sequence formulas is here to help you calculate the various types of problems related to GP like finding nth term, common ratio, the sum of the geometric series: X n = a + d(n−1) (We use "n−1" because d is not used in the 1st term)īy using the formula, we can find the summation of the terms of this arithmetic sequence. The general representation of arithmetic series is a, a + d, a + 2d.a + d(n−1)Īs per the rule or formula, we can write an Arithmetic Sequence as: Also, look at the below solved example and learn how to find arithmetic sequences manually.įind the sum of the arithmetic sequence of 2,4,6,8,10,12,14,16?Ī is the first term and d is the common difference By using this formula, we can easily find the summation of arithmetic sequences.įor practical understanding of the concept, go with our Arithmetic Sequence Calculator and provide the input list of numbers and make your calculations easier at a faster pace. If you substitute the value of arithmetic sequence of the nth term, we obtain S = n/2 * after simplification.Later, multiply them with the number of pairs.To solve the summation of a sequence, you need to add the first and last term of the sequence.The process to find the summation of an arithmetic sequence is easy and simple if you follow our steps.
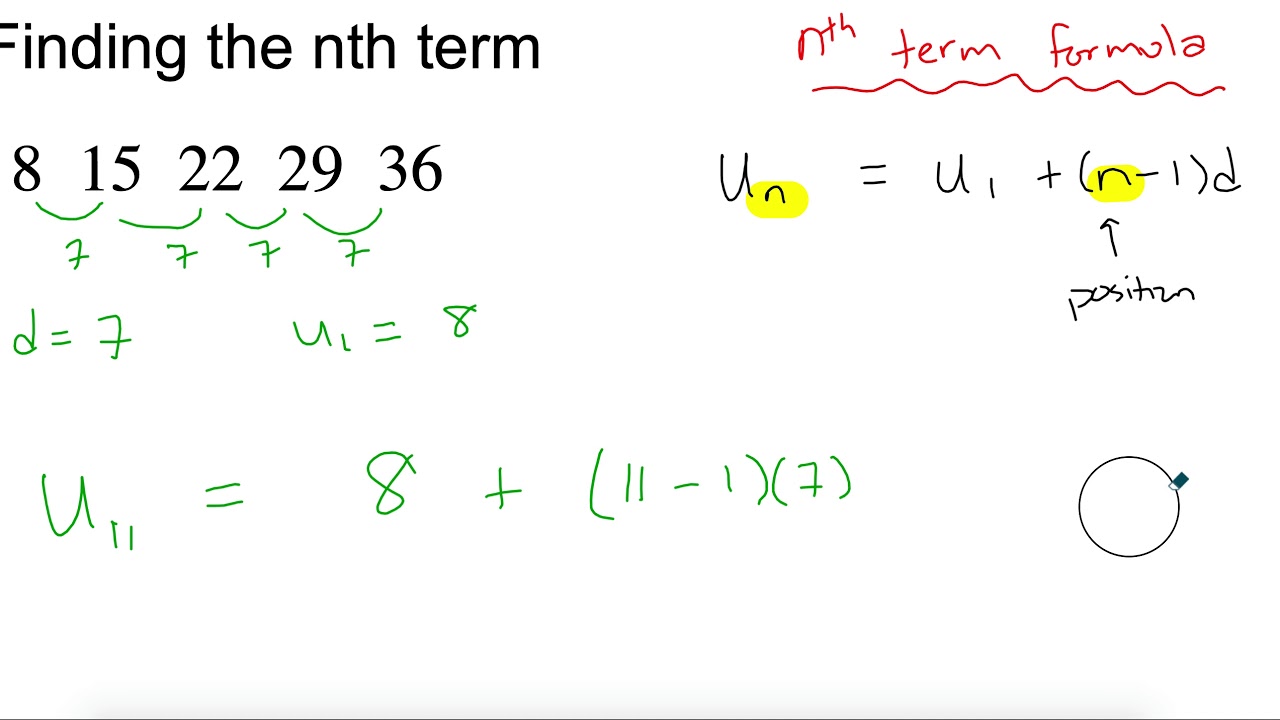
In case of the zero difference, the numbers are equal and there is no need to do further calculations. It is also used for calculating the nth term of a sequence. In case all the common differences are positive or negative, the formula that is applicable to find the arithmetic sequence is a n = a 1+(n-1)d. On a general note, it is sufficient if you add the n-1th term common differences to the first term. It takes much time to find the highest nth term of a sequence.


 0 kommentar(er)
0 kommentar(er)
